
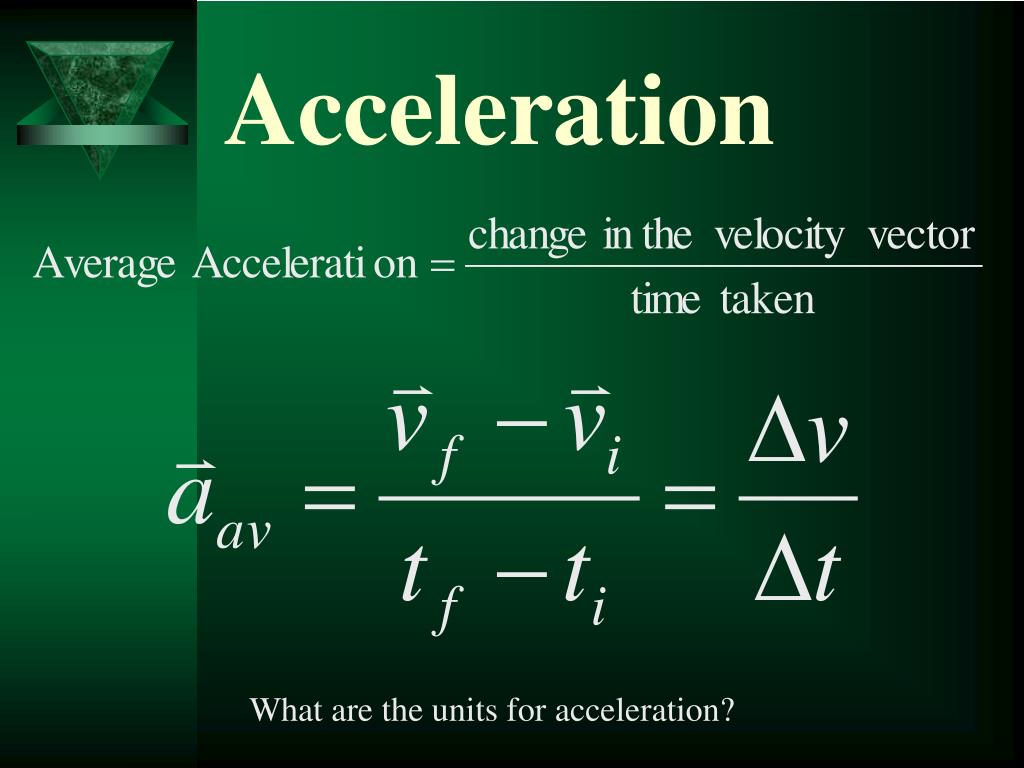
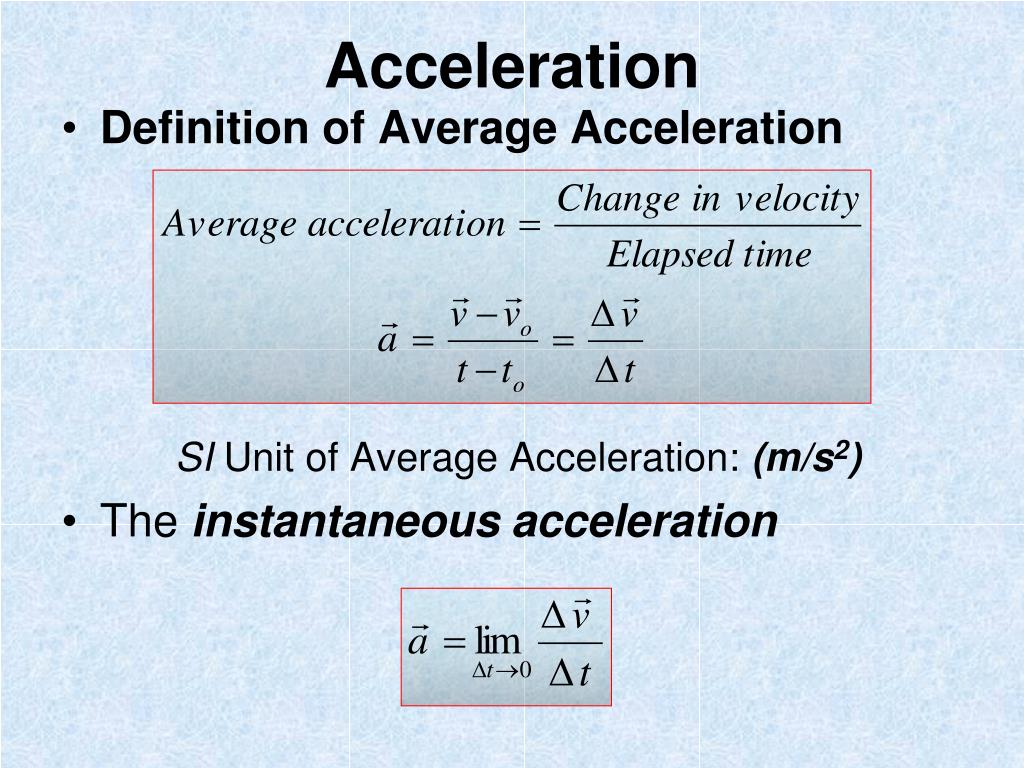
whether the object is speeding up or slowing down.The direction of the acceleration vector depends on two things: Since acceleration is a vector quantity, it has a direction associated with it. The (m/s)/s unit can be mathematically simplified to m/s 2. Since acceleration is a velocity change over a time, the units on acceleration are velocity units divided by time units - thus (m/s)/s or (mi/hr)/s.
For objects with a constant acceleration, the distance of travel is directly proportional to the square of the time of travel. Finally, if an object travels for four times the time, then it will cover 16 times (4^2) the distance the distance traveled after four seconds is 16 times the distance traveled after one second. If an object travels for three times the time, then it will cover nine times (3^2) the distance the distance traveled after three seconds is nine times the distance traveled after one second. As such, if an object travels for twice the time, it will cover four times (2^2) the distance the total distance traveled after two seconds is four times the total distance traveled after one second. The total distance traveled is directly proportional to the square of the time. Further analysis of the first and last columns of the data above reveal that there is a square relationship between the total distance traveled and the time of travel for an object starting from rest and moving with a constant acceleration. This discussion illustrates that a free-falling object that is accelerating at a constant rate will cover different distances in each consecutive second. Note: The ~ symbol as used here means approximately. These numbers are summarized in the table below. Given these average velocity values during each consecutive 1-second time interval, we could say that the object would fall 5 meters in the first second, 15 meters in the second second (for a total distance of 20 meters), 25 meters in the third second (for a total distance of 45 meters), 35 meters in the fourth second (for a total distance of 80 meters after four seconds). Our free-falling object would be constantly accelerating.
#ACCELERATION IUNIT FREE#
If we were to observe the motion of a free-falling object ( free fall motion will be discussed in detail later), we would observe that the object averages a velocity of approximately 5 m/s in the first second, approximately 15 m/s in the second second, approximately 25 m/s in the third second, approximately 35 m/s in the fourth second, etc. A falling object for instance usually accelerates as it falls. Since accelerating objects are constantly changing their velocity, one can say that the distance traveled/time is not a constant value.

Anytime an object's velocity is changing, the object is said to be accelerating it has an acceleration. In fact, the velocity is changing by a constant amount - 10 m/s - in each second of time. The velocity is changing over the course of time. The data at the right are representative of a northward-moving accelerating object. If an object is not changing its velocity, then the object is not accelerating. Acceleration has to do with changing how fast an object is moving. A person can be moving very fast and still not be accelerating. Yet acceleration has nothing to do with going fast. Sports announcers will occasionally say that a person is accelerating if he/she is moving fast. An object is accelerating if it is changing its velocity.


 0 kommentar(er)
0 kommentar(er)
